File Formats

This section documents the structure of Aion binary data files. The files described in this section can be created using the functions:

• SaveBooleanMatrix

• SaveComplexMatrix

• SaveIntegerMatrix

• SaveRealMatrix

and loaded into Aion using the functions:

• LoadBooleanMatrix

• LoadComplexMatrix

• LoadIntegerMatrix

• LoadRealMatrix

File Header

All binary data files use the same header format described in Table 54.

Table 54 Data File Header Format

Offset	Meaning	Encoding
5 - 0	Magic Number	0x49 0x4E 0x45 0x42 0x49 0x4E (ASCII for “INEBIN”)
6	Reserved	0x00
7	Matrix Type	ASCII: “B”, “Z”, “R”, or “C” representing boolean, integer, real, and complex matrices respectively.
11 - 8	Number Rows	4-byte little endian unsigned integer
15 - 12	Number Columns	4-byte little endian unsigned integer
16 - xx	Matrix Data	The application specific matrix data

Boolean Matrix Data Files

Boolean matrices are stored in a packed format with one bit per matrix entry. Entries are written in reading order across rows and then by row.

The zero based bit index into the matrix data can be calculated by:

\[i _ { index } = N _ c \left ( r - 1 \right ) + c - 1\]

Where \(i _ { index }\) is the bit index into the matrix data associated with a given matrix entry, \(N _ c\) indicates the number of matrix columns, \(r\) represents the one based row number, and \(c\) represents the one based column number.

The byte offset into the file where a given entry is stored can then be determined by:

\[i _ { offset } = \left \lfloor \frac{ i _ { index }}{8} \right \rfloor + 16\]

The bit offset into the byte is given by:

\[i _ b = \text{Remainder Of } \frac{i _ { index }}{8}\]

Bit offset 0 represents the least sigificant bit into each byte, bit offset 7 represents the most significant bit into each byte.

The matrix

\[\begin{split}A _ { i,j } \in \mathbb{B} \;\;\;\;\;\; A = \begin{bmatrix} 1 & 0 & 0 & 1 & 1 \\ 0 & 0 & 1 & 1 & 0 \\ 0 & 0 & 0 & 1 & 0 \end{bmatrix}\end{split}\]

would create the following binary file described in Table 55.

Table 55 Example Boolean Binary File

Offset	Contents	Representing	Meaning
0	0x49	“INEBIN”	File magic number.
1	0x4E
2	0x45
3	0x42
4	0x49
5	0x4E
6	0x00		Reserved
7	0x42	“B”	Indicates a boolean matrix.
8	0x03	3	Number rows
9	0x00
10	0x00
11	0x00
12	0x05	5	Number columns
13	0x00
14	0x00
15	0x00
16	0x99	10011 001	Matrix Data
17	0x21	10 00010 0

Below is sample code that writes a boolean matrix:

bool writeBooleanMatrix(
        const char* filename,
        unsigned    numberRows,
        unsigned    numberColumns,
        const bool* matrix
    ) {
    bool success = true;

    std::ofstream f(filename, std::ios::binary);
    if (f) {
        unsigned      i      = 6;
        std::uint8_t* buffer = new std::uint8_t[4096];

        std::memcpy(buffer, "INEBIN", 6);
        buffer[i++] = 0;
        buffer[i++] = 'B';

        buffer[i++] = static_cast<std::uint8_t>(numberRows      );
        buffer[i++] = static_cast<std::uint8_t>(numberRows >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 24);

        buffer[i++] = static_cast<std::uint8_t>(numberColumns      );
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 24);

        std::uint8_t  mask     = 1;
        unsigned long rowIndex = 0;
        std::uint8_t  v        = 0;
        while (success && rowIndex < numberRows) {
            unsigned long columnIndex = 0;
            while (success && columnIndex < numberColumns) {
                if (matrix[numberColumns * rowIndex + columnIndex]) {
                    v |= mask;
                }

                mask <<= 1;
                if (mask == 0) {
                    buffer[i++] = v;
                    v = 0;

                    mask = 1;

                    if (i == 4096) {
                        f.write(reinterpret_cast<const char*>(buffer), 4096);
                        success = static_cast<bool>(f);
                        i       = 0;
                    }
                }

                ++columnIndex;
            }

            ++rowIndex;
        }

        if (success) {
            if (mask != 1 || i != 0) {
                if (mask != 1) {
                    buffer[i++] = v;
                }

                f.write(reinterpret_cast<const char*>(buffer), i);
                success = static_cast<bool>(f);
            }
        }

        if (success) {
            f.close();
            success = static_cast<bool>(f);
        } else {
            success = false;
        }

        delete[] buffer;
    } else {
        success = false;
    }

    return success;
}

Integer Matrix Data Files

Integer matrices are stores in reading order across rows and then by row. Each entry is stored in signed little-endian format with 8-bytes per entry.

The zero based byte offset into the file where a given row/column entry starts is given by:

\[i _ { index } = 8 \left [ N _ c \left ( r - 1 \right ) + c - 1 \right ] + 16\]

The matrix

\[\begin{split}A _ { i,j } \in \mathbb{Z} \;\;\;\;\;\; A = \begin{bmatrix} 1 & 65536 & 0x010203040506 \\ -1 & -65536 & - 2 ^ {62} \end{bmatrix}\end{split}\]

would create the following binary file described in Table 56.

Table 56 Example Integer Binary File

Offset	Contents	Representing	Meaning
0	0x49	“INEBIN”	File magic number.
1	0x4E
2	0x45
3	0x42
4	0x49
5	0x4E
6	0x00		Reserved
7	0x42	“Z”	Indicates an integer matrix.
8	0x02	2	Number rows
9	0x00
10	0x00
11	0x00
12	0x03	3	Number columns
13	0x00
14	0x00
15	0x00
16	0x01	1	Matrix Data
17	0x00
18	0x00
19	0x00
20	0x00
21	0x00
22	0x00
23	0x00
24	0x00	65536
25	0x00
26	0x01
27	0x00
28	0x00
29	0x00
30	0x00
31	0x00
32	0x08	0x0102030405060708
33	0x07
34	0x06
35	0x05
36	0x04
37	0x03
38	0x02
39	0x01
40	0xFF	-1
41	0xFF
42	0xFF
43	0xFF
44	0xFF
45	0xFF
46	0xFF
47	0xFF
48	0x00	-65536
49	0x00
50	0xFF
51	0xFF
52	0xFF
53	0xFF
54	0xFF
55	0xFF
48	0x00	\(-2^{62}\)
49	0x00
50	0x00
51	0x00
52	0x00
53	0x00
54	0x00
55	0xC0

The sample code below writes an integer matrix to a binary file.

bool writeIntegerMatrix(
        const char*      filename,
        unsigned         numberRows,
        unsigned         numberColumns,
        const long long* matrix
    ) {
    bool success = true;

    std::ofstream f(filename, std::ios::binary);
    if (f) {
        unsigned      i      = 6;
        std::uint8_t* buffer = new std::uint8_t[4096];

        std::memcpy(buffer, "INEBIN", 6);
        buffer[i++] = 0;
        buffer[i++] = 'Z';

        buffer[i++] = static_cast<std::uint8_t>(numberRows      );
        buffer[i++] = static_cast<std::uint8_t>(numberRows >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 24);

        buffer[i++] = static_cast<std::uint8_t>(numberColumns      );
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 24);

        unsigned long rowIndex = 0;
        while (success && rowIndex < numberRows) {
            unsigned long columnIndex = 0;
            while (columnIndex < numberColumns) {
                long long v = matrix[numberColumns * rowIndex + columnIndex];
                *reinterpret_cast<std::int64_t*>(buffer + i) = v;
                i += sizeof(std::int64_t);

                if (i >= 4096) {
                    f.write(reinterpret_cast<const char*>(buffer), 4096);
                    success = static_cast<bool>(f);
                    i       = 0;
                }

                ++columnIndex;
            }

            ++rowIndex;
        }

        if (i != 0) {
            f.write(reinterpret_cast<const char*>(buffer), i);
            success = static_cast<bool>(f);
        }

        if (success) {
            f.close();
            success = static_cast<bool>(f);
        }

        delete[] buffer;
    } else {
        success = false;
    }

    return success;
}

Real Matrix Data Files

Real matrices are stores in reading order across rows and then by row. Each entry is stored as double precision values conforming with the IEEE 754-2008 standard as shown in Figure 227.

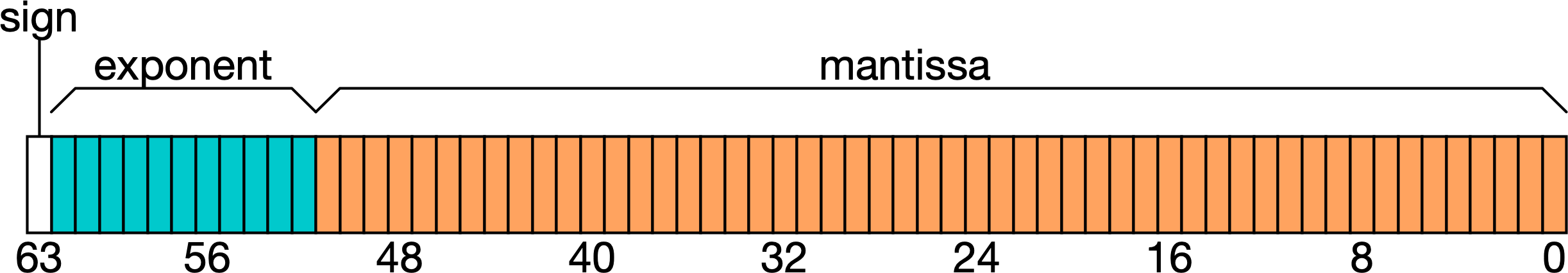
Figure 227 Real Number Format

Where the number value is represented by

\[(-1) ^ { sign } \left ( 1 + mantissa \right ) \times 2 ^ { exponent - 1023 }\]

The zero based byte offset into the file where a given row/column entry starts is given by:

\[i _ { index } = 8 \left [ N _ c \left ( r - 1 \right ) + c - 1 \right ] + 16\]

The matrix

\[\begin{split}A _ { i,j } \in \mathbb{R} \;\;\;\;\;\; A = \begin{bmatrix} 1 & 1.5 & 65536 \\ -1 & 0.375 & 0.0002 \end{bmatrix}\end{split}\]

would create the following binary file described in Table 57.

Table 57 Example Real Binary File

Offset	Contents	Representing	Meaning
0	0x49	“INEBIN”	File magic number.
1	0x4E
2	0x45
3	0x42
4	0x49
5	0x4E
6	0x00		Reserved
7	0x42	“R”	Indicates a real matrix.
8	0x02	2	Number rows
9	0x00
10	0x00
11	0x00
12	0x03	3	Number columns
13	0x00
14	0x00
15	0x00
16	0x00	1	Matrix Data
17	0x00
18	0x00
19	0x00
20	0x00
21	0x00
22	0xF0
23	0x3F
24	0x00	1.5
25	0x00
26	0x00
27	0x00
28	0x00
29	0x00
30	0xF8
31	0x3F
32	0x00	65536
33	0x00
34	0x00
35	0x00
36	0x00
37	0x00
38	0xF0
39	0x40
40	0x00	-1
41	0x00
42	0x00
43	0x00
44	0x00
45	0x00
46	0xF0
47	0xBF
48	0x00	0.375
49	0x00
50	0x00
51	0x00
52	0x00
53	0x00
54	0xD8
55	0x3F
56	0x2D	0.0002
57	0x43
58	0x1C
59	0xEB
60	0xE2
61	0x36
62	0x2A
63	0x3F

The sample code below writes a real matrix to a binary file.

bool writeRealMatrix(
        const char*   filename,
        unsigned      numberRows,
        unsigned      numberColumns,
        const double* matrix
    ) {
    bool success = true;

    std::ofstream f(filename, std::ios::binary);
    if (f) {
        unsigned      i      = 6;
        std::uint8_t* buffer = new std::uint8_t[4096];

        std::memcpy(buffer, "INEBIN", 6);
        buffer[i++] = 0;
        buffer[i++] = 'R';

        buffer[i++] = static_cast<std::uint8_t>(numberRows      );
        buffer[i++] = static_cast<std::uint8_t>(numberRows >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 24);

        buffer[i++] = static_cast<std::uint8_t>(numberColumns      );
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 24);

        unsigned long rowIndex = 0;
        while (success && rowIndex < numberRows) {
            unsigned long columnIndex = 0;
            while (columnIndex < numberColumns) {
                double v = matrix[numberColumns * rowIndex + columnIndex];
                *reinterpret_cast<double*>(buffer + i) = v;
                i += sizeof(double);

                if (i >= 4096) {
                    f.write(reinterpret_cast<const char*>(buffer), 4096);
                    success = static_cast<bool>(f);
                    i       = 0;
                }

                ++columnIndex;
            }

            ++rowIndex;
        }

        if (i != 0) {
            f.write(reinterpret_cast<const char*>(buffer), i);
            success = static_cast<bool>(f);
        }

        if (success) {
            f.close();
            success = static_cast<bool>(f);
        }

        delete[] buffer;
    } else {
        success = false;
    }

    return success;
}

Complex Matrix Data Files

Complex matrices are stores in reading order across rows and then by row. Each entry is stored as a pair of double precision values conforming with the IEEE 754-2008 standard as shown in Figure 227.

Real values are placed first in the file, followed by complex values.

The zero based byte offset into the file where a given row/column entry starts is given by:

\[i _ { index } = 16 \left [ N _ c \left ( r - 1 \right ) + c - 1 \right ] + 16\]

The matrix

\[\begin{split}A _ { i,j } \in \mathbb{R} \;\;\;\;\;\; A = \begin{bmatrix} 1+1,5i & 0.375+1.75i & 3 + 5i \\ 6+7i & 2 & 0.9375+31i \end{bmatrix}\end{split}\]

would create the following binary file described in Table 58.

Table 58 Example Complex Binary File

Offset	Contents	Representing	Meaning
0	0x49	“INEBIN”	File magic number.
1	0x4E
2	0x45
3	0x42
4	0x49
5	0x4E
6	0x00		Reserved
7	0x42	“C”	Indicates a complex matrix.
8	0x02	2	Number rows
9	0x00
10	0x00
11	0x00
12	0x03	3	Number columns
13	0x00
14	0x00
15	0x00
16	0x00	1	Matrix Data
17	0x00
18	0x00
19	0x00
20	0x00
21	0x00
22	0xF0
23	0x3F
24	0x00	1.5
25	0x00
26	0x00
27	0x00
28	0x00
29	0x00
30	0xF8
31	0x3F
32	0x00	0.375
33	0x00
34	0x00
35	0x00
36	0x00
37	0x00
38	0xD8
39	0x3F
40	0x00	1.75
41	0x00
42	0x00
43	0x00
44	0x00
45	0x00
46	0xFC
47	0x3F
48	0x00	3
49	0x00
50	0x00
51	0x00
52	0x00
53	0x00
54	0x08
55	0x40
56	0x00	5
57	0x00
58	0x00
59	0x00
60	0x00
61	0x00
62	0x14
63	0x40
64	0x00	6
65	0x00
66	0x00
67	0x00
68	0x00
69	0x00
70	0x18
71	0x40
72	0x00	7
73	0x00
74	0x00
75	0x00
76	0x00
77	0x00
78	0x1C
79	0x40
80	0x00	2
81	0x00
82	0x00
83	0x00
84	0x00
85	0x00
86	0x00
87	0x40
88	0x00	0
89	0x00
90	0x00
91	0x00
92	0x00
93	0x00
94	0x00
95	0x00
96	0x00	0.9375
97	0x00
98	0x00
99	0x00
100	0x00
101	0x00
102	0xEE
103	0x3F
104	0x00	31
105	0x00
106	0x00
107	0x00
108	0x00
109	0x00
110	0x3F
111	0x40

The sample code below writes a complex matrix to a binary file.

bool writeComplexMatrix(
        const char*                 filename,
        unsigned                    numberRows,
        unsigned                    numberColumns,
        const std::complex<double>* matrix
    ) {
    bool success = true;

    std::ofstream f(filename, std::ios::binary);
    if (f) {
        unsigned      i      = 6;
        std::uint8_t* buffer = new std::uint8_t[4096];

        std::memcpy(buffer, "INEBIN", 6);
        buffer[i++] = 0;
        buffer[i++] = 'C';

        buffer[i++] = static_cast<std::uint8_t>(numberRows      );
        buffer[i++] = static_cast<std::uint8_t>(numberRows >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberRows >> 24);

        buffer[i++] = static_cast<std::uint8_t>(numberColumns      );
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >>  8);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 16);
        buffer[i++] = static_cast<std::uint8_t>(numberColumns >> 24);

        unsigned long rowIndex = 0;
        while (success && rowIndex < numberRows) {
            unsigned long columnIndex = 0;
            while (columnIndex < numberColumns) {
                const std::complex<double>& v = matrix[
                    numberColumns * rowIndex + columnIndex
                ];

                *reinterpret_cast<double*>(buffer + i) = v.real();
                i += sizeof(double);
                *reinterpret_cast<double*>(buffer + i) = v.imag();
                i += sizeof(double);

                if (i >= 4096) {
                    f.write(reinterpret_cast<const char*>(buffer), 4096);
                    success = static_cast<bool>(f);
                    i       = 0;
                }

                ++columnIndex;
            }

            ++rowIndex;
        }

        if (i != 0) {
            f.write(reinterpret_cast<const char*>(buffer), i);
            success = static_cast<bool>(f);
        }

        if (success) {
            f.close();
            success = static_cast<bool>(f);
        }

        delete[] buffer;
    } else {
        success = false;
    }

    return success;
}